Blocky problem: The quest to find rectangles in a square

NEW YORK: Lisanne Taams, a student at Radboud University in the Netherlands, is working on a Ph.D. about, in her words, “computing motives of moduli stacks of vector bundles on stacky curves.” “It took me two years to even say that properly,” Taams said. But, she added, such heights of abstraction only elevated her delight as she recently spent time on a more concrete contemplation: counting the ways that a square can be divided into similar rectangles.

She found this geometric puzzle on Mathstodon, a community within the social network Mastodon. Created in the spring of 2017 by two mathematicians in England, Christian Lawson-Perfect and Colin Wright, registered accounts on Mathstodon totaled about 3,000 in September. Since then, with the Twitter exodus, the number has since increased to around 13,000. The puzzle was posted in December by John Carlos Baez, a mathematical physicist at the University of California, Riverside.

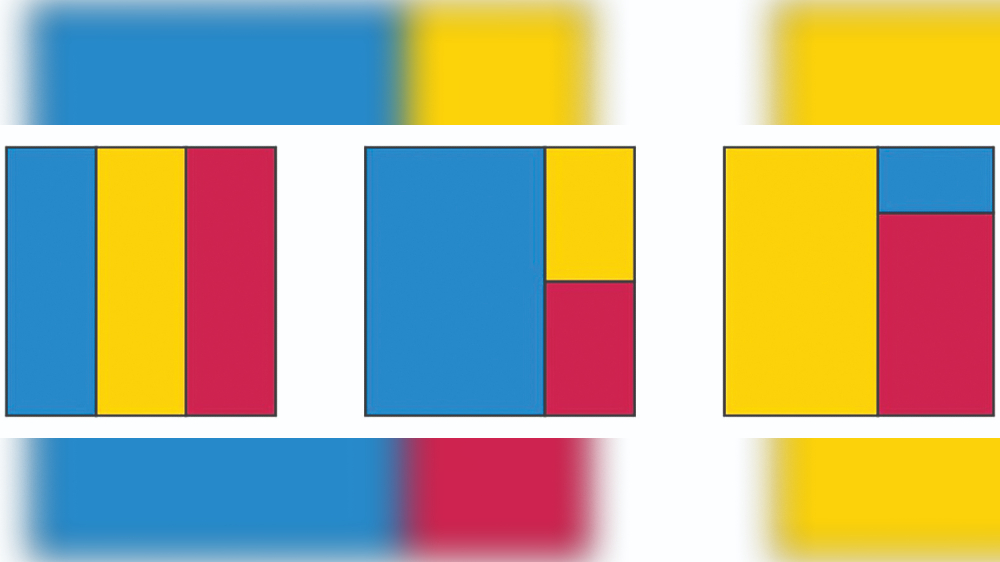
“There are three ways to divide a square into three rectangles with the same proportions!” Dr. Baez wrote. He illustrated the answer with three images that he borrowed from Wikipedia. In the image on the left, the rectangles are three times as long as they are wide, he explained in an email. In the middle image, the rectangles are one-and-a-half times as long as they are wide. “The third solution is trickier,” Dr. Baez said. The rectangles are “about 1.75487 times as long as they are wide, though one rectangle is turned around so it’s short and squat,” he added.

Dr. Baez noted that the number 1.75487 is of interest to mathematicians. “It’s the square of the ‘plastic ratio,’” he said, “which is a number that has a lot of properties similar to the more famous ‘golden ratio.’” Having laid that foundation, Dr. Baez asked his Mathstodon followers: “What if you chop a square into four similar rectangles? What proportions can they have?”

Among the first to take the bait was Rahul Narain, a computer scientist at the Indian Institute of Technology, Delhi. “I was on Mastodon before it was cool,” he says in his bio (he joined in December 2017). “And the fact that all the cool people are here now is a bit unsettling.”

Dr. Narain sketched out a systematic strategy for solving the puzzle, though he hoped someone else would carry it out. As he said in his reply to Dr. Baez, “I really have other things I need to work on right now, I can’t afford to get nerd-sniped any more than this!”

There turned out to be 11 solutions — 11 ways a square can be divided into four similarly proportioned rectangles. The solutions gradually accumulated with crucial input from Ian Henderson, an independent software developer in the Bay Area, and Daniel Piker in Bristol, England, who works as a design systems analyst developing software for architects at Foster + Partners. And a lot of other people also helped, Dr. Baez said. “That’s why it was fun.”

Taams found 11 solutions by hand and soon discovered that she had made a few mistakes. She then decided to let the computer do the work. She wrote software and generated some images. But when she checked the progress online, “I saw other people already had a lot more pictures,” Taams said.